Verify this identity (tan^2(x)1)/(1tan^2(x)) = 12cos^2(x) ~~~~~ It is done in 3 (three) easy steps 1 The numerator tan^2(x) 1 = = (1) 2 The denominator 1 tan^2(x) = = = (2) 3The Pythagorean identity tells us that no matter what the value of θ is, sin²θcos²θ is equal to 1 We can prove this identity using the Pythagorean theorem in the unit circle with x²y²=1Yes, sec 2 x−1=tan 2 x is an identity sec 2 −1=tan 2 x Let us derive the equation We know the identity sin 2 (x)cos 2 (x)=1 ——(i) Dividing throughout the equation by cos 2 (x) We get sin 2 (x)/cos 2 (x) cos 2 (x)/cos 2 (x) = 1/cos 2 (x) We know that sin 2 (x)/cos 2 (x)= tan 2 (x), and cos 2 (x)/cos 2 (x) = 1 So the equation (i) after substituting becomes

Answered 3 1 Tanx Tan 2x Sec 2x S Bartleby
Tan^2x-1 identity
Tan^2x-1 identity-You can put this solution on YOUR website!Verify the identity {eq}1 \tan^2x = \frac{\cos2x}{\cos^2x} {/eq} Identity An identity is an equation that holds true for any given variable value We have many commonly used trigonometric


How To Prove Csc 2x 1 Tan 2x 1 Is Equivalent To Cosec 2x Quora
= 2 cos2 x – 1 • Tangent tan 2x = 2 tan x/1 tan2 x = 2 cot x/ cot2 x 1 = 2/cot x – tan x tangent doubleangle identity can be accomplished by applying the same methods, instead use the sum identity for tangent, first • Note sin 2x ≠ 2 sin x;8/19/16 · (2cos^2x1)(1tan^2x)=1tan^2x Please Help?How to prove the identity (2cos^2x1)(1tan^2x)=1tan^2x Please Help?How to prove the identity This entry was posted in ATVs on August 19, 16 by Asher Burt Post navigation1/8/18 · Explanation This is readily derived directly from the definition of the basic trigonometric functions sin and cos and Pythagoras's Theorem Confirming that the result is an identity Yes, sec2 − 1 = tan2x is an identity
Identity tan (2x) Multiple Angle Identities Symbolab Identities Pythagorean Angle Sum/Difference Double Angle Multiple Angle Negative Angle Sum to Product Product to SumPeriodicity Identities, radians Periodicity Identities, degrees sin (x 2π) = sin xcsc (x 2π) = csc xsin (x 360°) = sin xcsc (x 360°) = csc xcos (x 2πThe trigonometric formulas like Sin2x, Cos 2x, Tan 2x are popular as double angle formulae, because they have double angles in their trigonometric functions For solving many problems we may use these widely The Sin 2x formula is \(Sin 2x = 2 sin x cos x\) Where x is the angle
You have seen quite a few trigonometric identities in the past few pages It is convenient to have a summary of them for reference These identities mostly refer to one angle denoted θ, but there are some that involve two angles, and for those, the two angles are denoted α and β The more important identities6/19/ · Yes, sec2−1=tan2x is an identity Why is cos an even function?Trigonometric Identities ( Math Trig Identities) sin (theta) = a / c csc (theta) = 1 / sin (theta) = c / a cos (theta) = b / c sec (theta) = 1 / cos (theta) = c / b tan (theta) = sin (theta) / cos (theta) = a / b cot (theta) = 1/ tan (theta) = b / a sin (x) = sin (x)



Cos2x Sin2x 1 1 Tan2x Sec2x Cot2x 1 Csc2x Cofunction



Double Angle Formulas Trigonometry Teachoo 2x 3x Formula Provi
3/25/15 · For questions 1 – 5, decide whether the equation is a trigonometric identity Explain your reasoning cos2 x(1 tan2 x) = 1 sec x tan x(1 – sin2 x) = sin x csc x(2sin x √2) = 0 cos2(2x) – sin2(2x) = 0 sin2 θ csc2 θ = sin2 θ cos2 θTan(x y) = (tan x tan y) / (1 tan x tan y) sin(2x) = 2 sin x cos x cos(2x) = cos 2 (x) sin 2 (x) = 2 cos 2 (x) 1 = 1 2 sin 2 (x) tan(2x) = 2 tan(x) / (1 · How to prove the trigonometry equation is an identity?



Ex 3 4 8 Find General Solution Of Sec 2 2x 1 Tan 2x Teachoo



Derive 1 Tan 2x Sec 2x And 1 Cot 2x Text Cosec 2x Geometrically Mathematics Stack Exchange
Verify the Identity tan(2x)^2sin(2x)^2cos(2x)^2=sec(2x)^2 Start on the left side Apply Pythagorean identity Tap for more steps Apply pythagorean identity Apply pythagorean identity Because the two sides have been shown to be equivalent, the equation is an identity is an identity1 cos A But sinA cosA =tanA and 1 cosA =secA (Notethat thedefinitionofthesecantofA is 1 cosA) Hence tan2 A1=sec2 A Thisisanotherimportantidentity Key Point tan2 A1=sec2 A Onceagain,returningto sin 2Acos A =1 wecandividethroughbysin2 A togive sin2 A sin 2A cos2 A sin A = 1 sin2 A But cosA sinA =cotA and 1 sinA =cosecASimplify trigonometric expressions Calculator online with solution and steps Detailed step by step solutions to your Simplify trigonometric expressions problems online with our math solver and calculator Solved exercises of Simplify trigonometric expressions
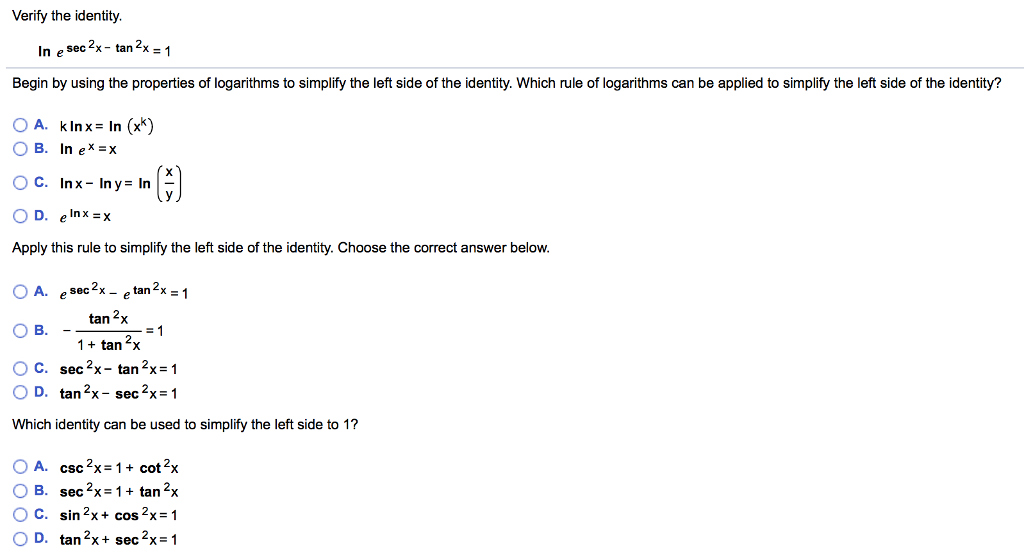


Solved Verify The Identity In Esec 2x Tan 2x 1 Begin By Chegg Com



1 Tan 2x 1 Cos 2x Sin 2x 2sin 4x 1 Sin 2x Trigonometric Identities Mcr3u Youtube
Siyavula's open Mathematics Grade 12 textbook, chapter 4 on Trigonometry covering Double angle identities$$2\cot4x = \cot2x \tan2x$$ Thank you in advance Thank you for the comments and hints I got an answer after many tries ;) Below is my answer Thank you $2cot4x = cot2x tan2x$ $2\frac{1}{tan 4x} = cot2x tan2x$ $2\frac{1}{\frac{2 tan 2x}{1 tan^2 2x}} = cot2x tan2x$X) 2 1 = 1 cos 2 x There aren't any terms or factors involving in this formula, so let's make up another one An equivalent formula for is math\implies\tan^2x=\dfrac {\sin^2x\sin^2x\cos^2x} {\sin /math Continue Reading


Tan2x ただの悪魔の画像



Tan 2x Csc 2x Tan 2x 1 Problem Solving Solving Identity
Equation 1 cot x = cos x/sin x equation 2 sec x = 1/cos x equation 3 csc x = 1/sin x equation 4 cot x = 1/tan x equation 5 sin 2 x cos 2 x = 1 equation 6 tan 2 x 1 = sec 2 x equation 7 1 cot 2 x = csc 2 x equation 8 cos (x y) = cos x cos y sin x sin y equation 9 sin (x y) = sin x cos y cos x sin y equation 10 cos (x) = cos x equation 11 cos (pi/2 x) = sin x equation 12=> `1 2*tan^2x` It is seen that `sec^4 x tan^4 x = 1 tan^2 x` is not an identity, instead `sec^4x tan^4x = 1 2*tan^2x` Approved by eNotes Editorial Team2 x I started this by making sec 1/cos and using the double angle identity for that and it didn't work at all in any way ever Not sure why I can't do that, but something was wrong Anyways I looked at the solutions manual and they magic out 1 tan x tan 2 x = 1 tan
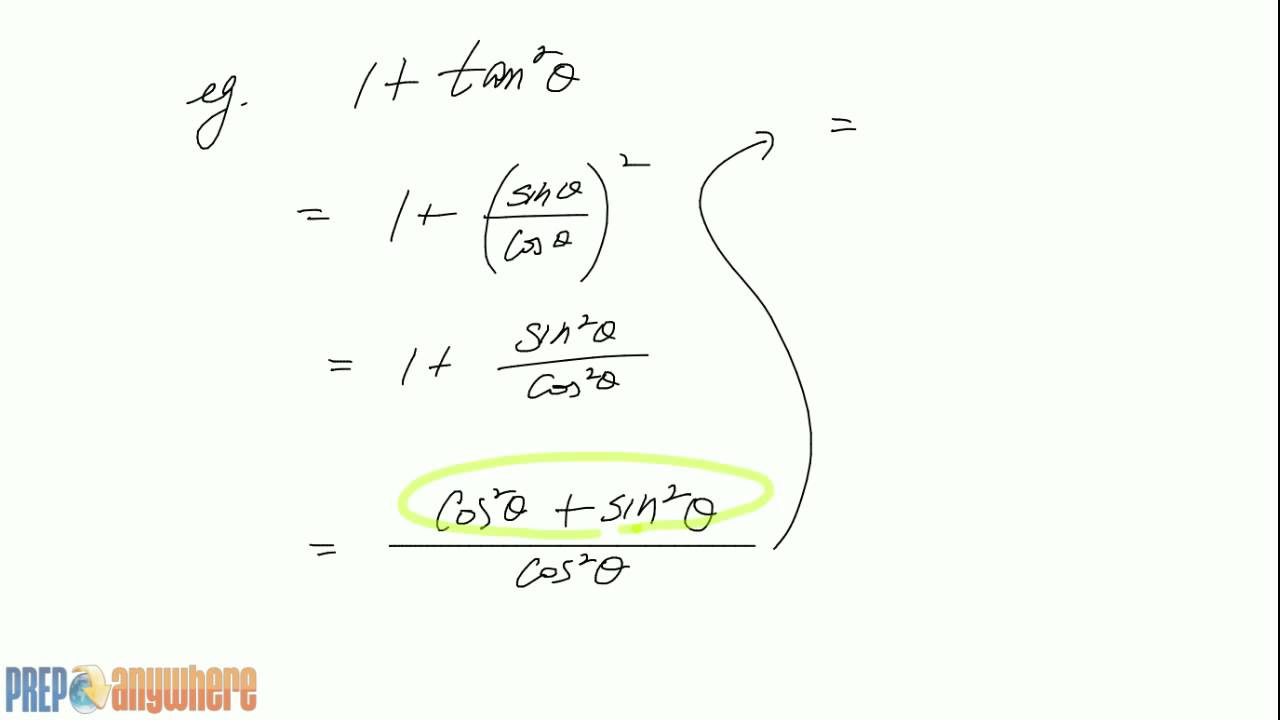


3 Simplifying Trig Expression 1 Tan 2x Youtube



Ln Sec 5 3 Key Pdf Ln 5 3 1 Verify The Identity A Math 1730 Name Key Sin 2 X 2sin X Cos X Cos 2 X Tan 2x 1 Cos 2



0 件のコメント:
コメントを投稿